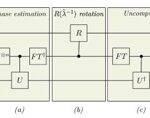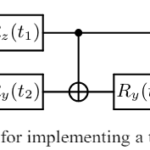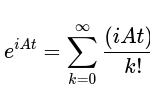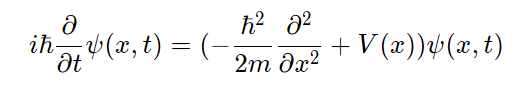
Note from Chat GPT
Discussions on angular momentum in quantum mechanics are crucial and deeply intertwined with many fundamental concepts. Let’s highlight some key points regarding angular momentum.
1. Angular Momentum Operators and Their Eigenvalues
In quantum mechanics, angular momentum is represented by operators. These operators act on the wave function, providing possible measured values (eigenvalues) of the system’s angular momentum. Operators for the total angular momentum magnitude and its z-component direction are commonly used.
2. Quantized Angular Momentum
The values of angular momentum are quantized, meaning they cannot take any arbitrary value but specific discrete values. This is evident from the angular momentum magnitude being ( \hbar\sqrt{l(l+1)} ) (where ( l ) is a non-negative integer, and (\hbar) is the reduced Planck constant) and its z-component being ( m\hbar ) (where ( m ) ranges from ( -l ) to ( +l )).
3. Conservation of Angular Momentum
Even in quantum systems, angular momentum is conserved unless acted upon by an external torque. This is similar to the conservation law in classical mechanics, though in quantum systems, it pertains to the probabilistic outcomes in a given state (wave function).
4. Spin and Orbital Angular Momentum
There are two main types of angular momentum in quantum mechanics: orbital angular momentum, which originates from the motion of particles, and spin angular momentum, an intrinsic property of particles. Although they are described by different operators and follow different rules, together they form the total angular momentum of a system.
5. Addition and Composition of Angular Momentum
In a system comprising multiple particles, the angular momenta of individual particles must be added to determine the total angular momentum. This process follows quantum rules, differing from classical vector addition, with the size and direction of the composite angular momentum determined by more complex rules.
6. Measurement of Angular Momentum and the Uncertainty Principle
The measurement of angular momentum is closely related to the quantum mechanical uncertainty principle. Precisely measuring the angular momentum component in one direction results in a loss of information about components in other directions. For example, accurately knowing the z-component of angular momentum means the components in the x and y directions are uncertain.