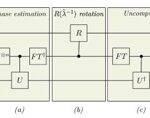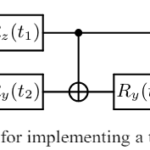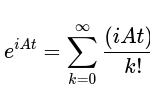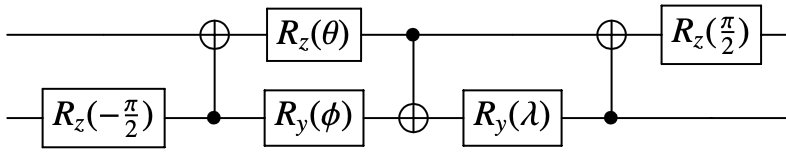
About
The SU(4) denotes a special unitary transformation of 4-dimensional complex vector space and has 15 independent generators, which must be Hermitian and traceless.
The generator of SU(4)
To construct the generator for SU(4), we take the tensor product of 2×2 Pauli matrices to generate 15 Hermitian traceless 4×4 matrices. Specifically, we consider the following combinations:
\begin{align}
\sigma_i \otimes \sigma_j \\
\sigma_i \otimes I \\
I \otimes \sigma_i
\end{align}Note that i, j are combination of x, y, z.
The next step is to check the Hermicity and Traceless of the generator of SU(4) above.
Checking Hermiticity
It is obvious that pauli matrices are Hermite.
\begin{align}
\sigma_x^ {\dagger} = \sigma_x \space, \space \sigma_y^\dagger = \sigma_y \space, \space \sigma_z^\dagger = \sigma_z \\
(\sigma_i \otimes \sigma_j)^\dagger = \sigma_i^\dagger \otimes \sigma_j^\dagger = \sigma_i \otimes \sigma_j
\end{align}Checking Traceless
And it is clear that trace of direct product of pauli equals to Trace product of Pauli matrices.
\operatorname{Tr}(\sigma_i \otimes \sigma_j) = \operatorname{Tr}(\sigma_i) \operatorname{Tr}(\sigma_j) = 0 \times 0 = 0